Triads

The three notes of a triad can always be arranged in thirds. Example 1 shows two triads, each written both melodically and harmonically:

Example 1. Two triads, shown melodically and harmonically.

The first triad is shown on three adjacent spaces, while the second triad is shown on three adjacent lines. A triad can always be “stacked” so that its notes are either on all lines or all spaces.

When a triad is stacked in its most compact form (measures 2 and 4 of Example 1), it looks like a snowperson. Example 2 shows several snowpeople:

A snowperson consists of a bottom, middle, and head. Likewise, a triad consists of a lowest note, a middle note, and an upper note. Colloquially, when a triad is stacked in this fashion it is sometimes called “snowperson form,” but non-colloquially it is known as root position; this is explored more in the the chapter titled Inversion and Figured Bass. The lowest note of a triad is called the root, the middle note is called the third, and the highest note is called the fifth. Example 3 shows this:

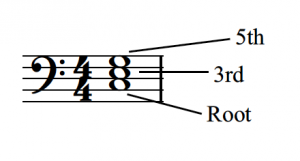
As you can see in Example 3, the third is so named because it is a generic third above the root, and the fifth is so named because it is a generic fifth above the root. The root is analogous to a snowperson’s bottom, the third to its middle, and the fifth to its head.

Triadic Qualities and Listening to Triads

There are four qualities of triad: major, minor, diminished, and augmented. Example 4a shows these four qualities of triad, each with a root of F and their quality of fifth labeled, while Example 4b shows these qualities with their quality of third labeled:

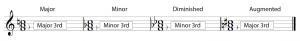
As seen in Example 4a and Example 4b, a major triad's fifth is perfect and its third is major, while a minor triad's fifth is perfect and its third is minor. A diminished triad's fifth is diminished and its third is minor, while an augmented triad's fifth is augmented and third is major. Major, minor, and diminished triads are more common in many genres of music, such as Classical and popular, which is why these triads are listed first in Example 4a and Example 4b. Augmented triads are less common in most Classical and popular music.

Example 5. Different qualities of triads.

Listen carefully to the different qualities of triad in Example 5. It is common to pair expressive qualities with triads when learning what they sound like. You might think of major triads as sounding “happy,” minor triads as “sad,” diminished triads as “scary,” and augmented triads as having a “fantasy” or “mystical” sound.

Chord Symbols

Chord symbols for triads often include the letter name of the triad’s root, the triad’s quality, and sometimes the pitch class that occurs in the bass voice, which is the lowest part (or voice) of a composition, regardless of what instrument or voice type is singing or playing that lowest note.

A chord symbol begins with a capital letter (and, if necessary, an accidental) denoting the root of the chord. That letter is followed by information about a chord’s quality:

• Major triad: no quality symbol is added
• Minor triad: lower-case “m”
• Diminished triad: lower-case “dim” or a degree sign “°”
• Augmented triad: lower-case “aug” or a plus sign “+”

For example, the chord symbols C, Cm, C^o, and C+ mean a C major triad, C minor triad, C diminished triad, and C augmented triad respectively. When the root of chords have accidentals you add these accidentals. For example, B♭m would be the chord symbol for a B♭-minor triad, while F♯^o would be the chord symbol for an F♯-diminished triad.

Finally, if a pitch class other than the chord root is the lowest note in the chord, a slash is added, followed by a capital letter denoting the pitch class in the bass (lowest) voice. Example 6 shows four triads with chord symbols:

Example 6. Four triads are shown with chord symbols.

As seen in Example 6, a triad that has a root of C and a major quality is shown as “C” in chord-symbol notation. A C minor triad, which consists of the notes C, E♭, and G, is written as “Cm” in chord-symbol notation if C were the lowest note. If E♭ were the lowest note of a C minor triad (see the third chord in Example 6), the chord symbol would be “Cm/E♭.” If G were the lowest note of a C minor triad (see the fourth chord in Example 6), the chord symbol would be “Cm/G.” This topic will be explored more in the chapter titled Inversion and Figured Bass.

Triad Qualities in Major and Minor

Triads can be built on any note of the major scale, as shown in Example 7:

Example 7. Qualities of triads in major keys.

Example 7 is in the key of G major. As you can see in Example 7, triads built on do, fa, and sol [latex](\hat1,\ \hat4,[/latex] and [latex]\hat5)[/latex] in major keys are major. This is shown in this example with the letter name of the triad’s root capitalized. Triads built on re, mi, and la [latex](\hat2,\ \hat3,[/latex] and [latex]\hat6)[/latex] are minor. This is shown with a lowercase letter “m” after the capital letter name of the triad’s root. Triads built on ti [latex](\hat7)[/latex] are diminished; this is shown with a superscript “^o” which you might know as the degree symbol. These triadic qualities do not change in different keys; in other words, the quality of a triad built on do [latex](\hat1)[/latex] is always major in major keys, no matter which major key a musical work is in.

Triads can be build on any note of the minor scale, as shown in Example 8:

Example 8. Qualities of triads built on the minor scale.

Note that Example 8 contains two triads built on sol [latex](\hat5)[/latex] and te/ti [latex](\downarrow\hat7[/latex]/[latex]\hat7)[/latex]—one without the raised leading tone (“natural minor”) and one with the raised leading tone (“ascending melodic minor”). As you can see in Example 8, triads built on do, fa, and sol [latex](\hat1,\ \hat4,[/latex] and [latex]\hat5)[/latex] (without the raised leading tone) are minor (shown with the lowercase “m”). Triads built on me, le, and te [latex](\downarrow\hat3,\downarrow\hat6,[/latex] and [latex]\downarrow\hat7)[/latex] (without the raised leading tone) are major. A triad built on sol [latex](\hat5)[/latex] with the raised leading tone is also major. Triads built on re and ti [latex](\hat2[/latex] and [latex]\hat7)[/latex] (with the raised leading tone) are diminished (shown with the superscript degree symbol).

Spelling Triads

To build a triad from a chord symbol, you need to be aware of a triad’s root and quality. Let’s say that, for example, we wanted to spell a major triad. We would complete the following steps:

1. Draw the root on the staff.
2. Draw notes a third and fifth above the root (i.e. draw a snowperson).
3. Think of (or write down) the key signature of the triad’s root.
4. To spell a major triad, apply any accidentals from the key signature if notes in that key signature appear in the triad.
5. For a minor, diminished, or augmented triad, add additional accidentals to alter the chord’s third and/or fifth when appropriate.

Example 9 shows this process for a D major triad:

Example 9. Drawing a D major triad in four steps.

The process for writing this triad is as follows:

1. First, the note D, the chord’s root, is drawn on the staff.
2. Second, a snowperson is drawn—an F and A, the notes a generic third and a fifth above the D.
3. Third, the key signature of D major has been recalled. D major has two sharps, F♯ and C♯.
4. Fourth, a sharp (♯) has been added to the left of the F, because F♯ is in the key signature of D major. No C♯ was necessary because there is no C in the chord.

Let’s complete this process for an A♭ minor triad (A♭m), as seen in Example 10:

Example 10. Drawing an A♭ minor triad in five steps.

The process for writing this triad is as follows:

1. First, the note A♭ is written because it is the root of the triad.
2. Second, a snowperson is drawn; in other words, the notes C and E are added because they are a generic third and fifth respectively above A♭.
3. Third, the key signature of A♭ major is recalled. A♭ major has four flats, B♭, E♭, A♭, and D♭. Fourth, E♭ is added, because it is in the key signature of A♭ major. No B♭ or D♭ are needed, because those notes aren’t in an A♭ triad.
4. Now we have successfully spelled an A♭-major triad (A♭, C, and E♭).
5. Minor triads contain a minor third, which is one half-step smaller than a major third. Therefore, our final step is to lower the chord’s third (the C) by a half-step (to a C♭). Now we have an A♭ minor triad (A♭, C♭, and E♭).

Don’t forget that diminished triads have a minor third and a diminished fifth, meaning you have to lower both the third and the fifth by a half-step from a major triad. An augmented triad has a major third and an augmented fifth, so its fifth must be raised by a half-step from a major triad.

Identifying Triads, Doubling, and Spacing

Triads are identified according to their root, quality, and inversion; however, inversion is not discussed until the chapter Inversion and Figured Bass. Example 11 shows a triad for the process of identification:

Example 11. A triad for identification.

You can identify triads in three steps:

1. Identify and write its root.
2. Imagine the major key signature of its root.
3. Identify and write its quality.

To identify this triad:

1. First identify and write its root, which is the lowest note in its most compact form—in this case D.
2. Now you can identify and write its quality. To do this, you will need to imagine the major key signature of its root. The key of D major has two sharps, F♯ and C♯.
3. In this triad, the F is sharp, matching the major key signature. We would correctly identify this as a D major triad.

Example 12 shows a second triad for the process of identification:

Example 12. A second triad for identification.

To identify this triad:

1. First we identify and write its root, which is C♯.
2. Next we can identify and write its quality. We imagine the key signature of C♯ major, which has seven sharps (every note is sharp).
3. Therefore, E and G would be sharp in a C♯ major key. Instead, both of these notes have been lowered by a half-step; therefore, this triad is diminished. We would correctly identify this triad as a C♯^o triad.

If the bottom note of a triad has an imaginary key signature (because there is a double accidental that applies to it), use enharmonic equivalence to respell the triad. The process for this will be the same as that outlined in the last section of Intervals.

Because musicians accept octave equivalence, the doubling of notes does not affect a triad’s identification. Example 13 shows several different triads with octave doublings and their correct identification:

Example 13. Two triads with doublings have been identified.

As you can see, the identification of these triads is the same, regardless of octave doublings, even when more than one clef is used.

Notes can also be spaced further apart, which is called open spacing. Example 14 shows two triads in open spacing:

Example 14. Two triads in open spacing.

As you can see in Example 14, all of the notes of each triad appear, but they are widely spaced across several different octaves within a grand staff.

Doublings and open spacing can be combined, as seen in Example 15:

Example 15. Two triads with doublings in open spacing.

Neither of these factors will affect how you identify these triads. In order to identify triads with doublings and open spacing, you need to either imagine or write the notes into a triad in closed spacing without any doublings.

• Introduction to Chords (musictheory.net)
• Triad Introduction (Robert Hutchinson)
• What is a Triad? (YouTube)
• Building Triads (YouTube)
• Constructing Triads (Columbia)
• Lead-sheet Symbols (Robert Hutchinson)
• Triad Identification Practice Tool (teoria)
• Chord Ear Training (musictheory.net)
• Triad Ear Training (Tone Dear)
• Triad Ear Training (teoria)

1. Triad Identification (.pdf, .pdf, .pdf, .pdf, .pdf, .pdf, .pdf, .pdf)
2. Triad Construction (.pdf), pp. 2, 4, 6, 7 (.pdf), p. 9 (.pdf)

1. Triads Assignment #1 (.pdf, .mcsz)
2. Triads Assignment #2 (.pdf, .mcsz)
3. Triads Assignment #3 (.pdf, .mcsz)